
GOD’S CHESS RATING
February 8th, 2021If God were playing chess, would his ELO rating be bounded?
Against a similar being a perfect player would always draw, or win/lose depending on color and whether chess is a theoretical win/draw e.g. for white. If we assume that perfect play leads to draw then God would never lose but sometimes draw assuming other beings are capable of perfect games; God would therefore have a bounded ELO, as any win percentage less than 1.0 against a given opponent directly translates to a finite ELO difference .
In fact a ‘Shakespeare monkey’ playing random moves would play a perfect game every once in a while , somewhere around one in (1/20)^20 games if there are ~20 possible moves per turn and ~20 turns per game for one side.
And for sure if chess is a win (e.g. for white) then there’s a theoretical limit to the ELO of a perfect player, since gods would either win or lose to each other 50/50 (depending on whether they have to play white/black) , and would have better odds against mortals (and would lose occasionally to mortals who happened to play a perfect game as white, ensuring that the population of perfect players can’t drift away in rating from the population of imperfect players).
The expected outcome as function of ELO difference looks like this:

Now knowing there’s some ELO for a perfect player, what is it?
If one could determine a fraction of perfect games that e.g. the world champion M. Carlsen can play against God, then it would be possible to relate God’s rating to Carlsen’s. One thing that jumps to mind is, as a player approaches perfection the loss fraction should go down even if the win fraction doesnt rise – so I’d expect more draws and fewer wins/losses assuming play against approximately equal opponents – if chess is a draw. And likewise if chess is a win then the draw fraction should drop with increasing ELO, hitting 0 at a perfect player’s rating.
So we can look at the draw rate (humans and engines included below) as function of ELO rating:
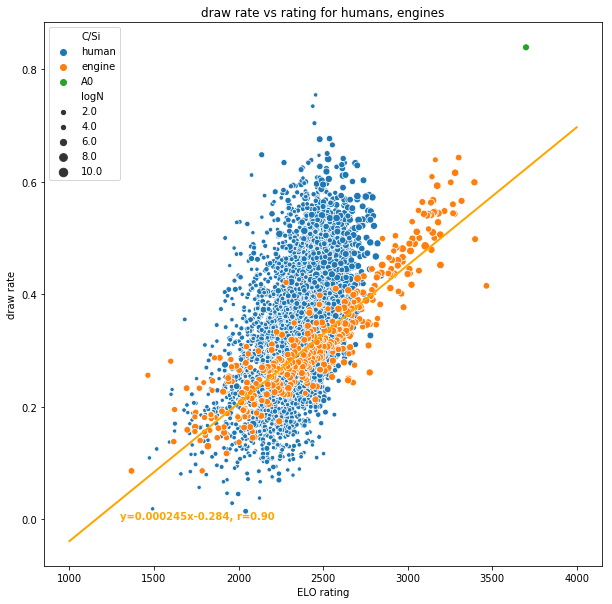
This tells us a few things: 1. it would appear that chess is a theoretical draw; and 2. a lower bound for a perfect player’s rating.
The line hits y=draw_rate=1 at rating=5237 which is a stab at a lower bound for a perfect player’s rating. One imagines that this curve should asymptote to 1 however so the lower limit isn’t necessarily anywhere close to the actual value.
In case you’re curious, it looks like computer ratings over time are strikingly linear, which may not be surprising if rating is linear with search depth; the number of positions per depth being exponential, and the speed of computers likewise increasing exponentially with time.

If everything holds till then , computers will hit the 5200 rating in the year 2059.
Quantum computers would radically change this , I suppose. In fact the long-held notion that chess is ‘unsolvable’ may be wrong in light of the quantum computer.